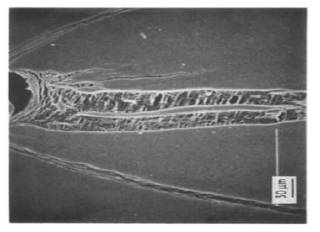
MOLECULAR DYNAMICS OF FRACTURE IN POLYMERS

Crazing is a precursor to failure in many glassy amorphous polymers. The susceptibility of a polymer to deform by crazing depends on its structure and entanglement density. Crazing involves the formation of fibrils bridging two layers of undeformed polymer, which subsequently elongate and fail leading to the formation or elongation of a crack. Hence crazes behave as load bearing structures delaying fracture and hence contribute greatly to the fracture toughness of a polymer. The study of the nucleation, growth and failure of crazes will help in preventing crazing or improving the fracture toughness of a polymer.

Substantial studies have been done on the phenomenon of crazing. Experimental studies have shown that a typical craze has remarkable dimensions, is around 20 nm in thickness initially and grow to 3mm before failure.

Initiation of crazes occurs near preexisting flaws or embedded particles under appropriate stress conditions. This phase of crazing is the least understood aspect because the length scales are inaccessible to experimental studies. Most of the available literature is based on stress bias conditions and require the knowledge of local stresses. The growth phase is relatively well understood. The fibrils elongate by pulling in polymer from a semi deformed layer above known as active layer. The growth is at constant volume fraction of fibril, by disentanglement and scission of chains in the active zone, causing flow into the fibril. The dimensions of fibrils and spacing between fibrils have been determined experimentally. The fibrils rupture or disentangle subsequently causing breakdown of fibril. But a clear understanding of the ending of the growth process and failing of fibrils is not established.

Molecular simulations offer tools to examine physical phenomenon closely but are limited by length and time scales. However the insights gained from MD can be used to interpret the phenomenon. This will also help in establishing the validity of assumptions made to study the crazing parameters. The most important thing is to design the simulations such that they are representative of a true craze in spite of the obvious limitations of MD with regards to the time and length scales. This involves a proper choice of force field, structure of the polymer, simulation parameters like sample size, deformation conditions such as strain rate etc.

The present work aims to study the initiation, growth and failure phenomena in crazes in order to gain further insight into experimentally observed facts. Further, we aim to extract sufficient information from our MD studies in order to be able to build a so called cohesive zone model that can be used to enrich a continuum model of the polymer.